Qui aura 20 en maths ?
💯 Le grand concours 100% Terminale revient fin janvier 2026 à l'ESIEA Paris !Découvrir →
Nouveau
🔥 Découvre nos fiches d'exercices gratuites avec corrections en vidéo !Accéder aux fiches →
Lire des limites dans un tableau de variation - Exercice 1
3 min
5
Lire des limites dans un tableau de variation.
Question 1
Soit le tableau de variation de la fonction ci-dessous : 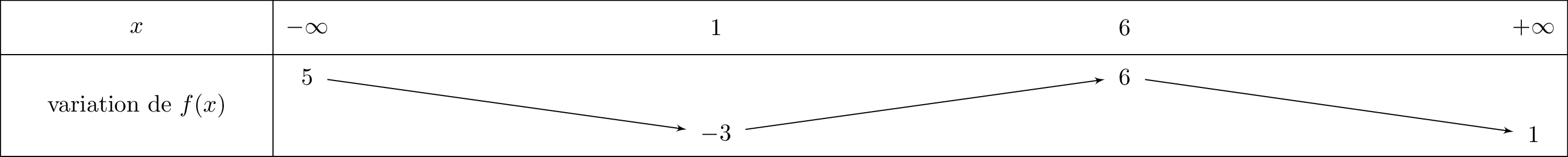

Quelles sont les limites de en et . Interpréter ce résultat graphiquement?
Correction
Si où est une valeur finie alors la fonction admet une asymptote horizontale d'équation
Si où est une valeur finie alors la fonction admet une asymptote horizontale d'équation
Si où est une valeur finie alors la fonction admet une asymptote horizontale d'équation
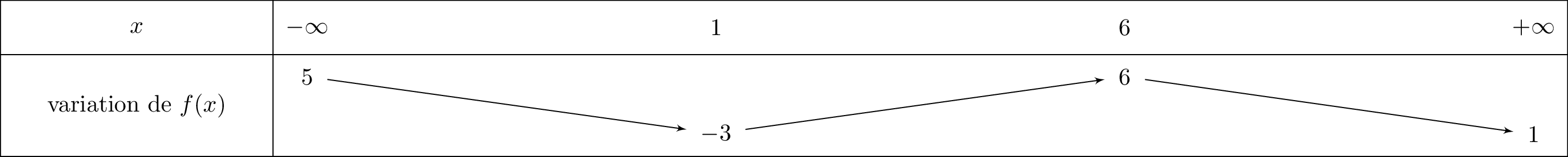
et
Comme alors la fonction admet une asymptote horizontale d'équation
Comme alors la fonction admet une asymptote horizontale d'équation
Signaler une erreur
Aide-nous à améliorer nos contenus en signalant les erreurs ou problèmes que tu penses avoir trouvés.
Connecte-toi ou crée un compte pour signaler une erreur.