Nouveau
🤔 Bloqué sur un exercice ou une notion de cours ? Échange avec un prof sur le tchat !Découvrir →
Exercices types : ère partie - Exercice 4
15 min
25
Soit la fonction définie sur par .
On note sa courbe représentative dans un repère orthonormal .
On note sa courbe représentative dans un repère orthonormal .
Question 1
Déterminer les limites de la fonction en et en .
Correction
. On pose , ainsi .
Enfin, par composition
D'où : .
On pose , ainsi .
Par composition :
. On pose , ainsi .
Enfin, par composition
D'où .
On pose , ainsi .
Par composition :
On en déduit que la courbe admet une asymptote horizontale d'équation au voisinage de .
Question 2
Etudier le sens de variation de .
Correction
On reconnait la forme
On a et
Ainsi
Or pour tout réel , on sait que et que .
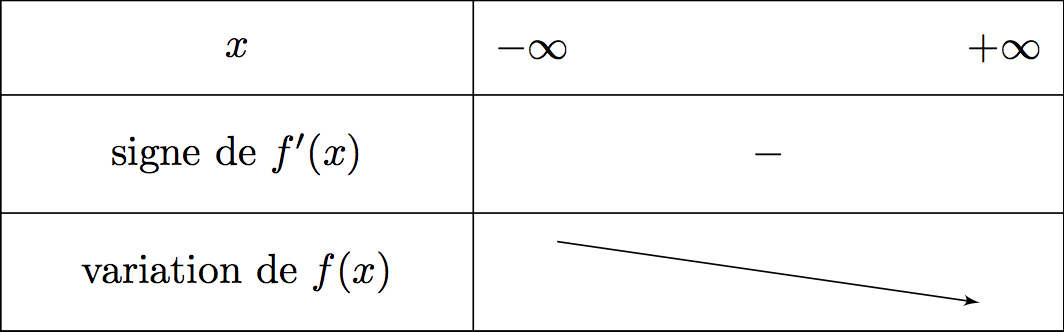
De ce fait, est strictement négative donc est strictement décroissante sur
On en déduit le tableau de variation donné ci-dessous :
On a et
Ainsi
Or pour tout réel , on sait que et que .
De ce fait, est strictement négative donc est strictement décroissante sur
On en déduit le tableau de variation donné ci-dessous :

Question 3
Démontrer que pour tout nombre réel : .
Correction
On va factoriser l'expression par .
Il vient alors que :
On substitue cela dans l'expression de .
D'où :
Comme alors
Signaler une erreur
Aide-nous à améliorer nos contenus en signalant les erreurs ou problèmes que tu penses avoir trouvés.
Connecte-toi ou crée un compte pour signaler une erreur.