Nouveau
🤔 Bloqué sur un exercice ou une notion de cours ? Échange avec un prof sur le tchat !Découvrir →
Déterminer des modules à l'aide de la définition - Exercice 3
6 min
15
Question 1
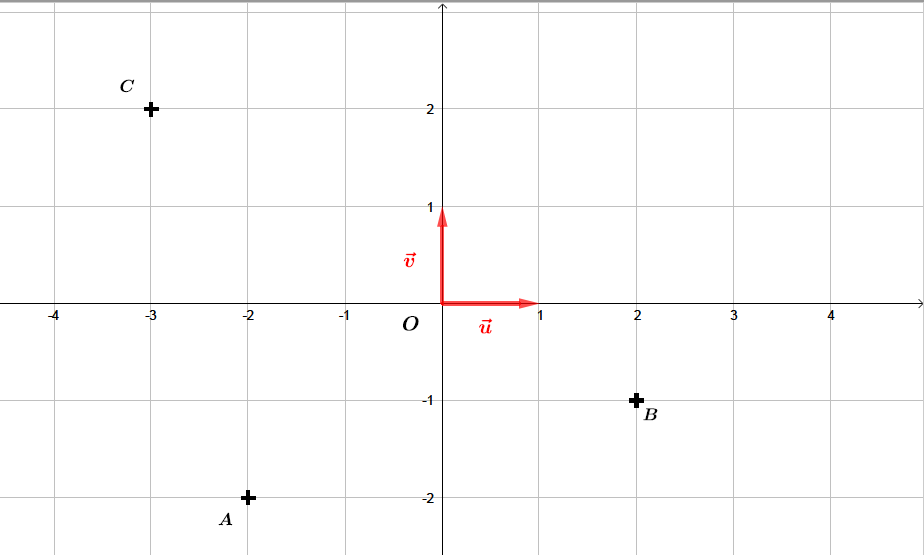
On travaille dans le plan complexe rapporté au repère orthonormal direct . On donne les points ; et dont les affixes respectives sont ; et
Placer les points , et .
Correction
Question 2
Déterminer les distances ; et .
Correction
- Soient et deux points d'affixe respective et .
Question 3
Que peut-on en déduire quant à la nature du triangle ?
Correction
nous venons de montrer que . Le triangle est donc isocèle en .
On vérifie que :
et que
D'après la réciproque du théorème de Pythagore, le triangle est rectangle en .
Finalement, le triangle est un triangle rectangle isocèle en .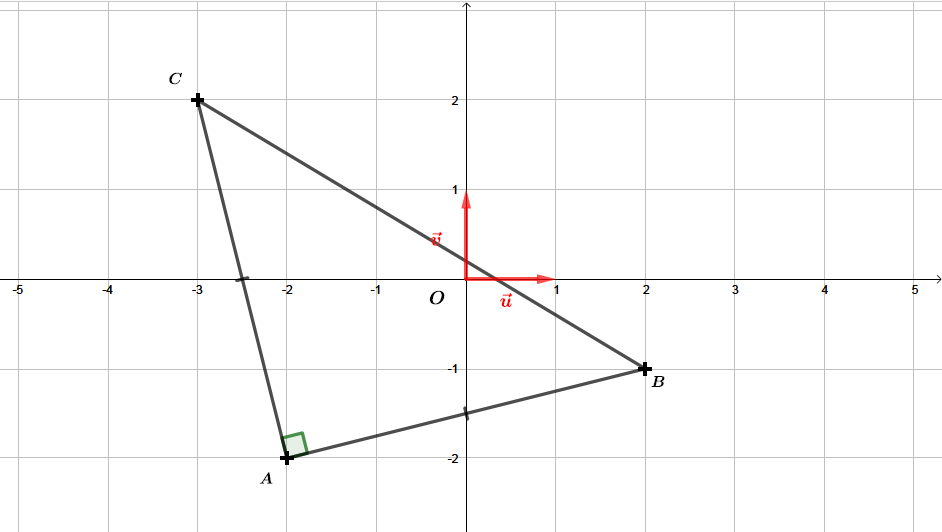
On vérifie que :
et que
D'après la réciproque du théorème de Pythagore, le triangle est rectangle en .
Finalement, le triangle est un triangle rectangle isocèle en .

Signaler une erreur
Aide-nous à améliorer nos contenus en signalant les erreurs ou problèmes que tu penses avoir trouvés.
Connecte-toi ou crée un compte pour signaler une erreur.