Qui aura 20 en maths ?
💯 Le grand concours 100% Terminale revient fin janvier 2026 à l'ESIEA Paris !Découvrir →
Nouveau
🔥 Découvre nos fiches d'exercices gratuites avec corrections en vidéo !Accéder aux fiches →
On continue encore les D.L. simples ! - Exercice 1
45 min
65
Déterminer les D.L. des fonctions proposées.
Question 1
Déterminer le D.L., en zéro et à l'ordre 5, de .
Correction
On a :
Or on a :
Comme alors on a le droit de poser . On a alors, en se limitant à l'ordre :
Puis, par intégration, on trouve que :
Or . Ainsi :
Or on a :
Comme alors on a le droit de poser . On a alors, en se limitant à l'ordre :
Puis, par intégration, on trouve que :
Or . Ainsi :
Question 2
Déterminer le D.L., en zéro et à l'ordre 5, de .
Correction
On a :
Or on a :
Comme alors on a le droit de poser . On a alors, en se limitant à l'ordre :
Soit encore :
Puis, par intégration, on trouve que :
Or . Ainsi :
Or on a :
Comme alors on a le droit de poser . On a alors, en se limitant à l'ordre :
Soit encore :
Puis, par intégration, on trouve que :
Or . Ainsi :
Question 3
Déterminer le D.L., en zéro et à l'ordre 5, de .
Correction
On a :
Or on a :
Comme alors on a le droit de poser . On a alors, en se limitant à l'ordre :
Soit encore :
Puis, par intégration, on trouve que :
Or . Ainsi :
Or on a :
Comme alors on a le droit de poser . On a alors, en se limitant à l'ordre :
Soit encore :
Puis, par intégration, on trouve que :
Or . Ainsi :
Question 4
Déterminer le D.L., en zéro et à l'ordre 5, de .
Déterminer le D.L., en zéro et à l'ordre 5, de .
Correction
On sait que pour tout réel, on a :
Soit encore :
Or, on a :
Donc :
Par soustraction, on trouve que :
Par simplification, on trouve que :
Finalement :
Soit encore :
Or, on a :
Donc :
Par soustraction, on trouve que :
Par simplification, on trouve que :
Finalement :
Question 5
Par une division suivant les puissances croissantes, déterminer le D.L., en zéro et à l'ordre 5, de .
Correction
On sait que :
Avec :
Donc, on va effectuer la division euclidienne du développement limité de par le développement limité de , selon les puissance croissante de . On a alors en limitant au termes de puissance :
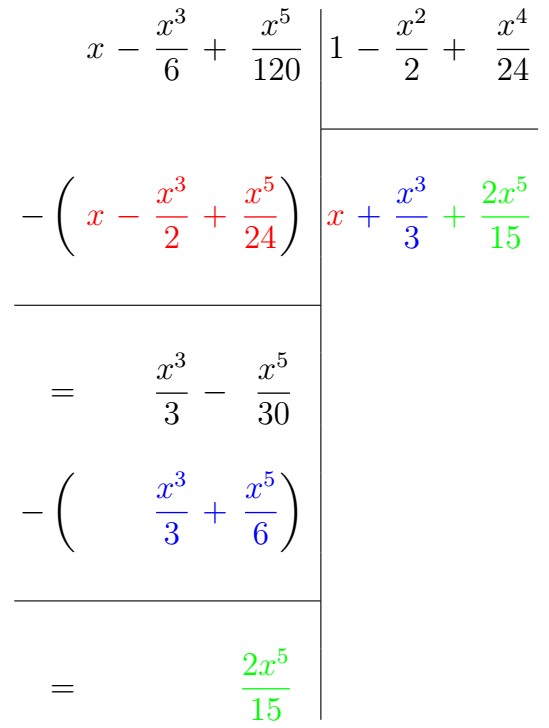
On en déduit donc que :
Avec :
Donc, on va effectuer la division euclidienne du développement limité de par le développement limité de , selon les puissance croissante de . On a alors en limitant au termes de puissance :

On en déduit donc que :
Signaler une erreur
Aide-nous à améliorer nos contenus en signalant les erreurs ou problèmes que tu penses avoir trouvés.
Connecte-toi ou crée un compte pour signaler une erreur.