Qui aura 20 en maths ?
💯 Le grand concours 100% Terminale revient fin janvier 2026 à l'ESIEA Paris !Découvrir →
Nouveau
🔥 Découvre nos fiches d'exercices gratuites avec corrections en vidéo !Accéder aux fiches →
Asymptote - Exercice 1
45 min
70
Un exemple de calcul asymptotique.
Question 1
On pose, pour , .
Déterminer un développement limité de l'expression en .
Correction
Lorsque alors . On a alors :
Comme , on a alors :
Ainsi :
Comme , et que également, on a alors :
Ce qui nous donne, à l'ordre :
Donc :
Soit :
En développant :
Soit encore :
Ainsi, on en déduit que :
On obtient alors :
Finalement :
Comme , on a alors :
Ainsi :
Comme , et que également, on a alors :
Ce qui nous donne, à l'ordre :
Donc :
Soit :
En développant :
Soit encore :
Ainsi, on en déduit que :
On obtient alors :
Finalement :
Question 2
Déterminer l'équation de la parabole, notée , asymptotique à en .
Correction
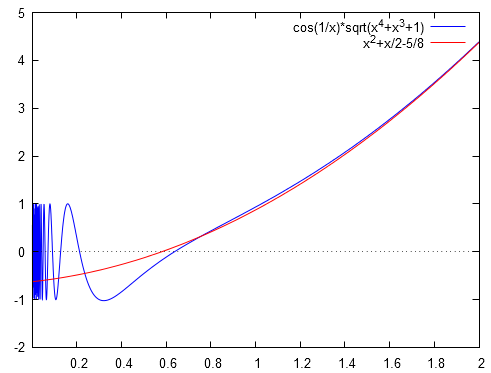
Le passage de lorsque nous donne l'équation de la parabole asymptotique à .
D'après la question précédente, on en déduit que l'équation de est :
On peut vérifier ceci sans peine par les représentations graphiques associées à et . On a alors :
D'après la question précédente, on en déduit que l'équation de est :
On peut vérifier ceci sans peine par les représentations graphiques associées à et . On a alors :

Question 3
Déterminer, en , la position relative de par rapport à .
Correction
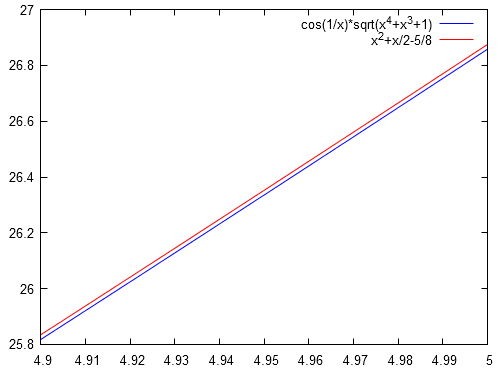
On a :
Ce qui revient à dire que lorsque on a :
Soit encore (lorsque ) :
Ainsi l'asymptote est de .
Graphiquement, cela se vérifie très aisément. On a :
Ce qui revient à dire que lorsque on a :
Soit encore (lorsque ) :
Ainsi l'asymptote est de .
Graphiquement, cela se vérifie très aisément. On a :

Signaler une erreur
Aide-nous à améliorer nos contenus en signalant les erreurs ou problèmes que tu penses avoir trouvés.
Connecte-toi ou crée un compte pour signaler une erreur.